Ce document présente un résumé écrit à la main du chapitre probabilité pour les sections bac sciences expérimentales, bac économie et gestion, bac technique, bac informatique et bac mathématiques.

Matière: Maths

Chapitre: Probabilité

Sections: Toutes les sections scientifiques

Contenu: Résumé et exercices corrigés

Probabilité : Résumé et Exercices corrigés

Dérombrement: Page Fb: WaelDocuments.com

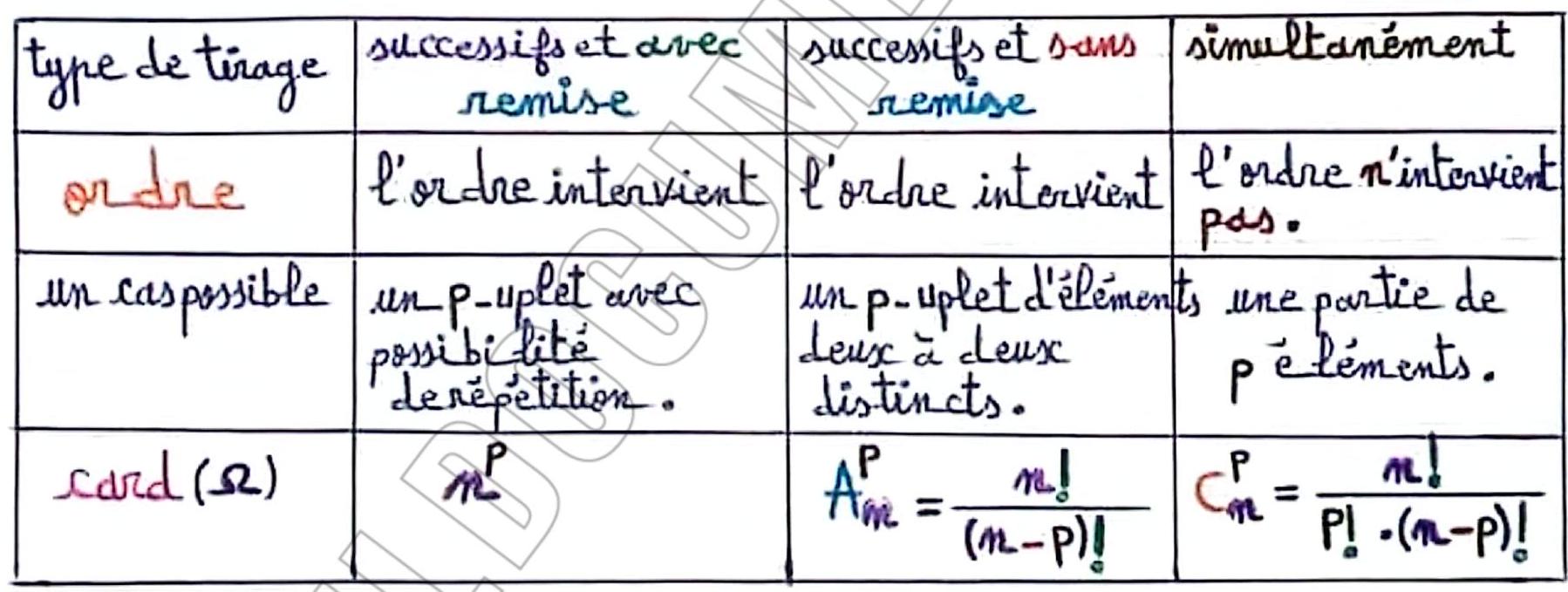
(1) le cardinal d’un enssemble fini E désigne le nombre d’éléments de $E$, on note card (E).

AELDOCUMENTS

(2) soit $A$ et $B$ dewse parties de $E$

$$
\operatorname{card}(A \backslash B)=\operatorname{cand}(A)-\operatorname{card}(A \cap B)
$$

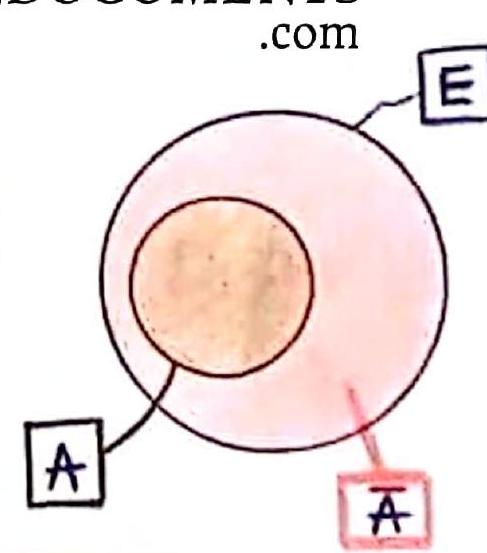
$\operatorname{cand}(\bar{A})=\operatorname{card}(E)-\operatorname{card}(A)$

$\bar{A}:$ le complémentaire de $A$.

$$
\operatorname{cand}(A \cup B)=\operatorname{card}(A)+\operatorname{card}(B)-\operatorname{cand}(A \cap B)
$$

si $A$ et $B$ ne sont pas disjoints

AELDOCUMENTS لديف: لديفر

Rappel $=$

(5T0P) soit $A$ et $B$ deax enssembles finis.

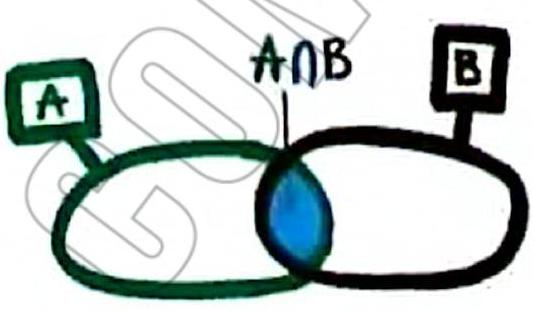
leurs intersections $(A \cap B)$ qui se dit $A$ inter $B$, est l’enssemble des éléments qui appartiennent a lofois à $A$ et à $B$ (2)

(570P)

Les exssembles A et B sont diets disjoints

lorsqu’ils r’ont aucur élement commur.

$A$ et $B$ disfoints $\Leftrightarrow A \cap B=\varnothing$

Q) AELDOCUMENTS

STOP soit $A$ et $B$ deux enssembles finis.

leurs reunion (AUB), qui se dit $A$ unior $B$, est l’enssemble dont les éléments appertionnent à $A$ oul $B$.

• si $A$ et $B$ sont disjoints a lors $\operatorname{card}(A \cup B)=\operatorname{card}(A)+\operatorname{card}(B)$
• si $A$ et $B$ ne sent pas disjoints alors =

$$
\operatorname{cand}(A \cup B)=\operatorname{cand}(A)+\operatorname{cdrd}(B)-\operatorname{cand}(A \cap B)
$$

Arrangement

on appelle arrangement de péléments d’un enssemble $E$ à néléments. ( card $(E)=n$ ) tout $p$-uplet d’éléments deux ci deux distincts del le nombre d’arrangement de $p$ clements de $E$ est:

$$
A_{n}^{p}=\frac{n !}{(n-p) !}
$$

AELDOCUMENTS

on appelle peamutatior de $n$ iléments de $E$ tout $n$-uplet d’éléments deux ì deux destincts de $E$.

le nombre de permutation de $E$ égul à $A_{n}^{n}=n !=n \cdot(n-1) \cdot(n-2) \ldots$

$$
\ldots \times 2 \times 1
$$

suit $E=\left{a_{1,} a_{2}, a_{3}, \ldots, a_{n}\right}$ un enssemble à $n$ éléments et $p$ un entier maturel non nul.

le nombre de p-uplet de E est le nombre d’application (produit cartésien) estégal a $\pi^{P}$.
on appelle combindison de $p$ éléments de E, toute partie ap éliments distincts de $E$.

le nombre de combinaison de $p$ éléments de $E$ est

$$
C_{n}^{p}=\frac{A_{n}^{p}}{p !}=\frac{n !}{P ! \cdot(n-p) !}
$$

Tirage de $p$ boules parmi $n$ boules:

يمكنكم شراء كتب ورقيّة

للباكالوريا من 2009 إلى إلى 2023 بالاصلاح الرّسمي

بأسوام طيّارة على صفحتنا ( WaelDocuments.com

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

Page Fb: WaelDocuments.com

Probabilité تـفـمعe بأسمأ طريقة 8
$\star$
0
0 Excercice de

Q0 tirage simultanné $\xrightarrow{\text { dboximi }} C_{m}^{p}=\frac{n !}{p ! x(n-p) !}$

أ formule probabilité d’un eventerment $p$ (evenemint) $=\frac{\text { card (EVenement) }}{\text { card }(\Omega)}$

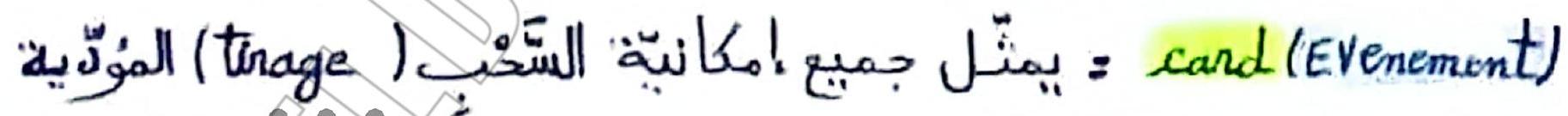
AELDOCUMENTS . Evenement لتح

5ده محتد من الكوبرات من مجمعي الكريرات الهوجودة بالقدندوت.
exemple d’application =

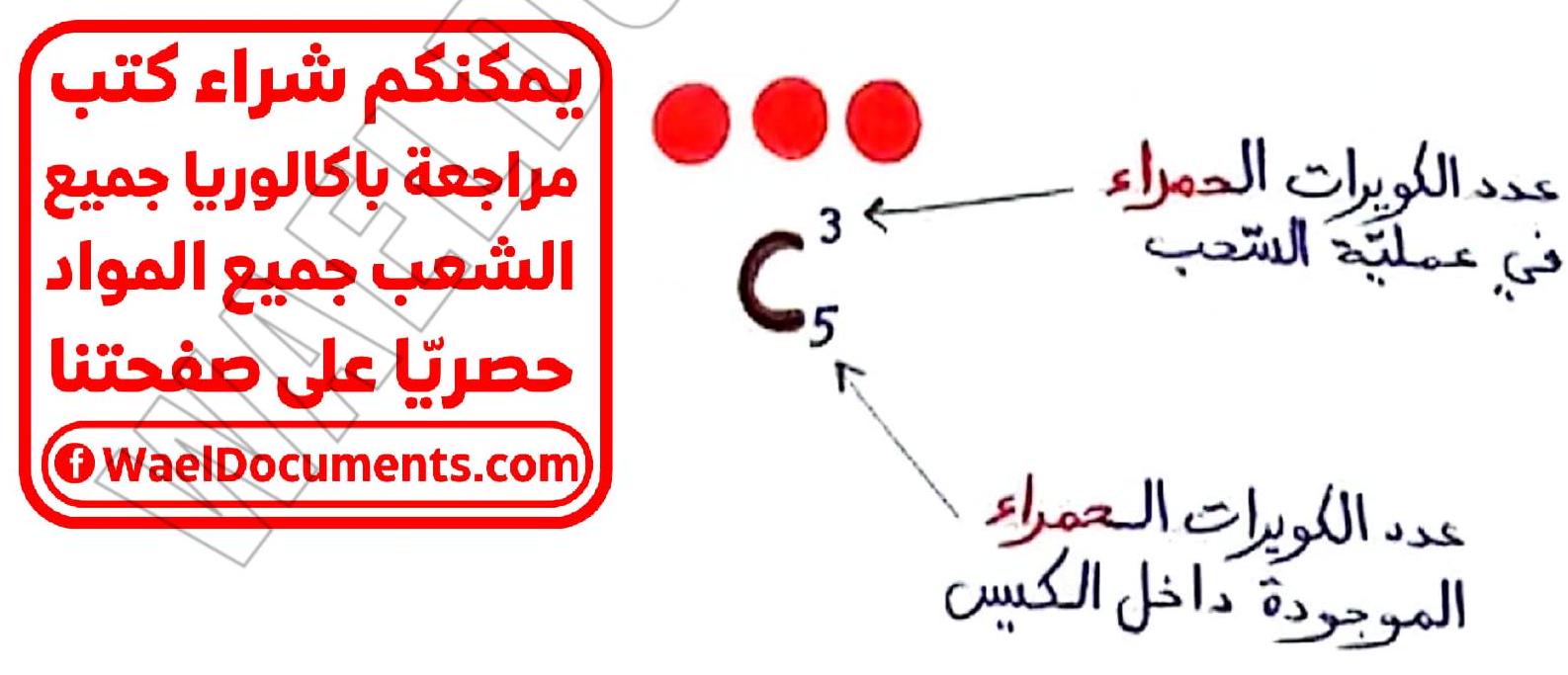
un stac contient 5 boules rouges, 4 boules noireset 3 boulesvert

on tire simultannément 3 boules de $l$ sac.

soient les evenements suivants =

A: «obtenir 3 borles a méme coulears»-

$B:$ : 0 btonir 3 boules de coaleurs différents».

$C$ : «obtenir wurams ure baule rouge».

prababilite d’in

Evenciment

لأحنسابن

card(everrement) êventment

• تدتّنّ

= formule 4

$$
p\left(\text { evenement) }=\frac{\text { cand (eventount) }}{\text { fard }(\Omega)}\right.
$$
$1 \%$ calculer $p(A), p(B)$ et $p(c)$ calcul de $p(A)=$

$A$ obtenir 3 boules de même couleur $0^{00042-150}$ إملانيّات البّحب المؤدّية للدمول على 3 boules de même cialeno

00 on 000 on 000

$$
\operatorname{card}(A)=C_{5}^{3}+C_{4}^{3}+C_{3}^{3}
$$

$$
=\text { = }
$$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

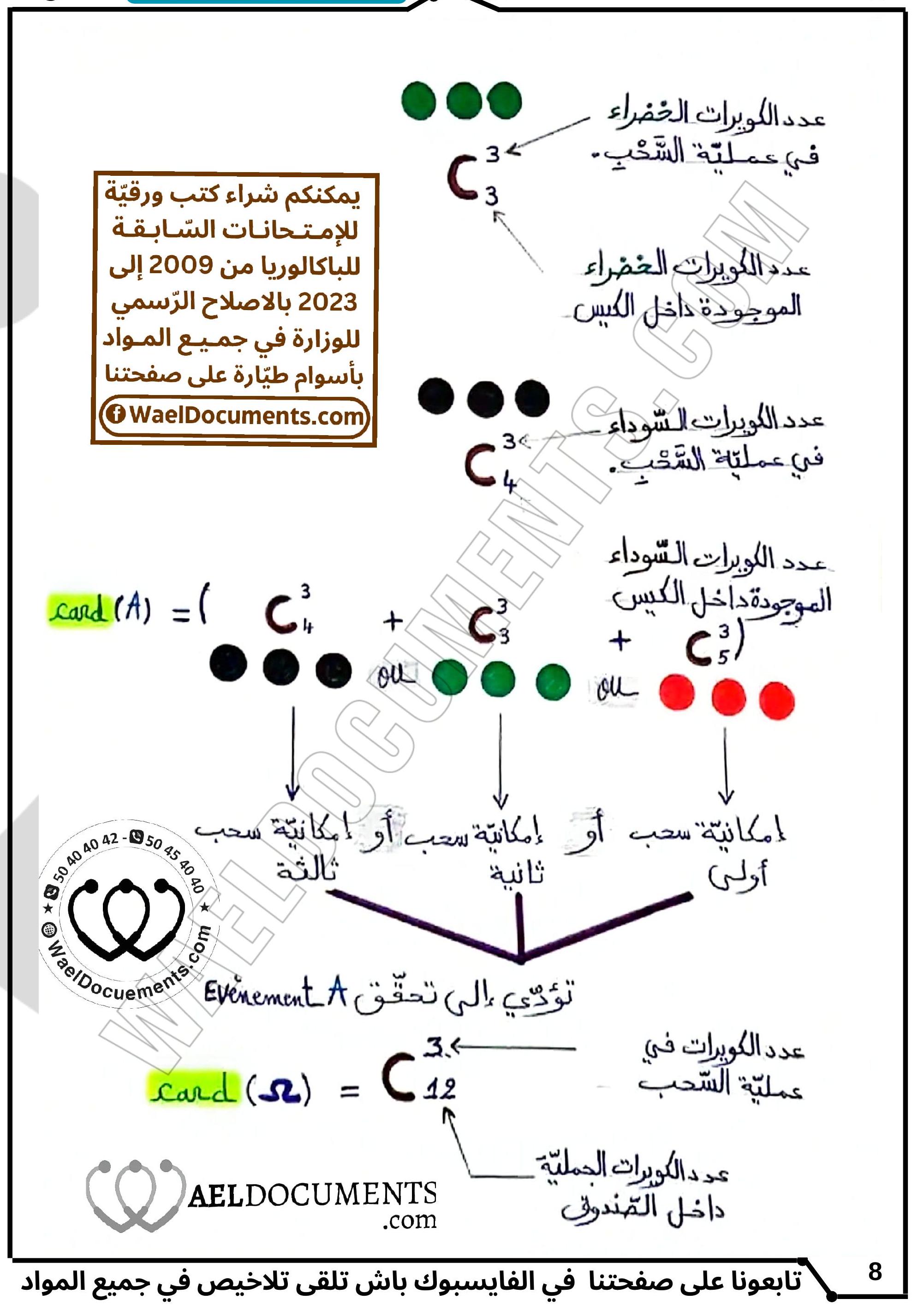
$C:$ : obtenir all moins une boule rouge»

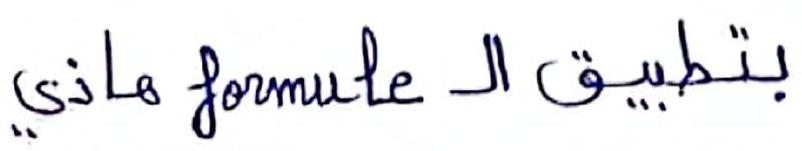
$$
p(c)=1-p(\bar{C})
$$

• p(c)

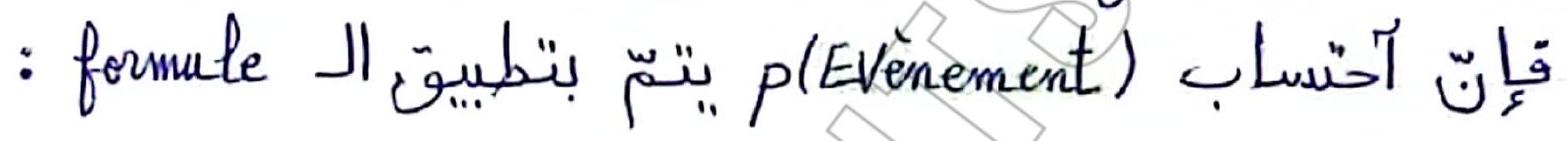
$$
p \text { (EVènement) }=1<p \text { (EVènement) }
$$

وردت ني.تعريفه

AELDOCUMENTS

« all moins » كلم

$\vec{c}=<$ obtenir aucune boule rouge »0

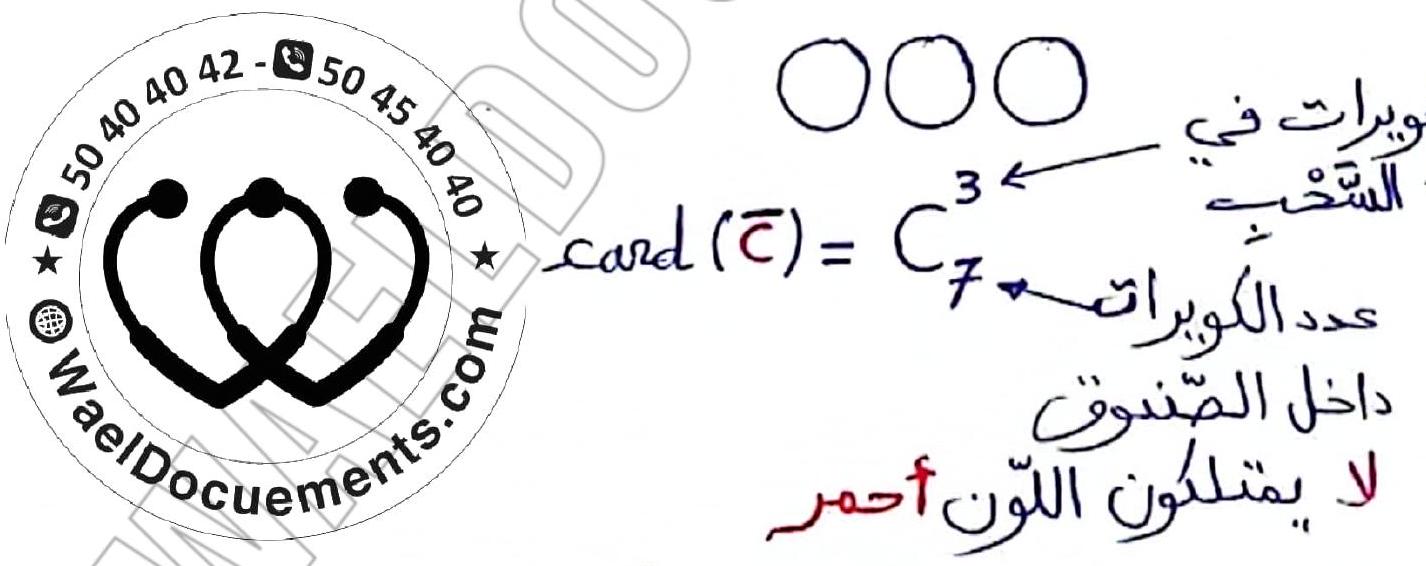
$$
\begin{aligned}
& p(c)=1-p(\bar{c}) \
& =1-\frac{\operatorname{card}(\bar{C})}{\operatorname{card}(\Omega)} \
& =1-\frac{C_{7}^{3}}{C_{19}^{3}}
\end{aligned}
$$

$$
\begin{aligned}
p(A)=\frac{\operatorname{card}(A)}{\operatorname{cand}(\Omega)} & =\frac{C_{4}^{3}+C_{3}^{3}+C_{5}^{3}}{C_{12}^{3}} \
& =\frac{4+1+10}{220} \
& =\frac{15}{220}
\end{aligned}
$$

calcul de $p(B)=$

$B$ «ottenir 3 boules de couleurs différents»

3 boules de couleurs différents

AELDOCUMENTS

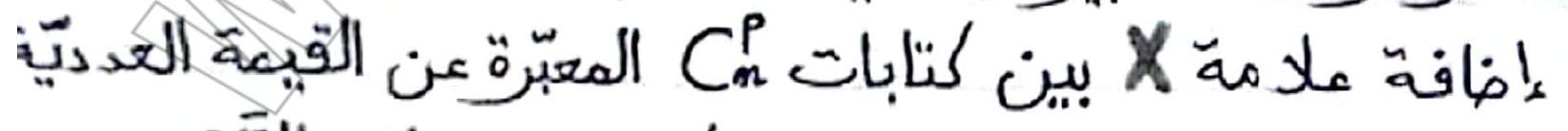
$$
\text { كدد الكوبرات }
$$

البّونيء دأخل الليسم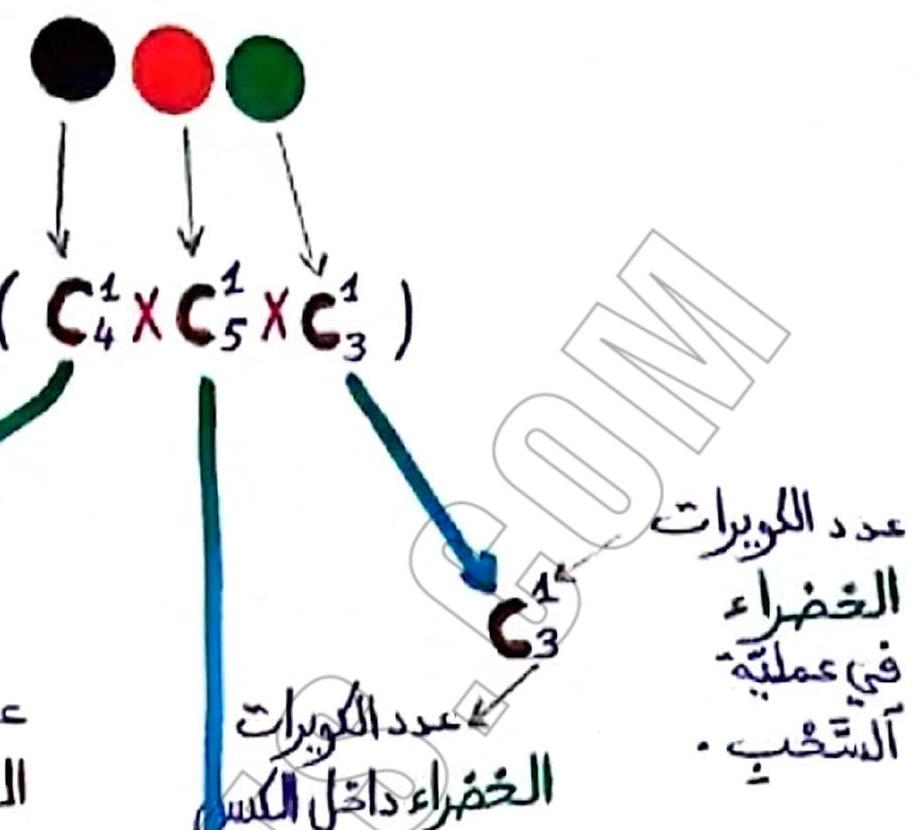

$$
\operatorname{card}(B)=\left(\boldsymbol{C}{4}^{1} \times \boldsymbol{C}{5}^{1} \times \mathbf{C}_{3}^{1}\right)
$$

$$
\begin{aligned}
& \text { عدد الكوبرات } \
& \text { السوداء في } \
& \text { كملتَمَ الشَتْتُ }
\end{aligned}
$$

$$
p(B)=\frac{\operatorname{cand}(B)}{\operatorname{cand}(\Omega)}=\frac{C_{4}^{1} \times C_{5}^{1} \times C_{3}^{1}}{C_{12}^{3}}
$$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

Page Fb: WaelDocuments.com

EXERCICE: Probabilité

un sac contient trois jetons rouges, deux jetons verts et un jeton noir.

1\% on tire simultannément deux jetons du sac. seit les évènements suivants:

$A=\ll$ obtenir deux jetous de même couleur»

B: «abtenir all moins un jetons vert»

1\%

a) Vérifier que $p(A)=\frac{4}{15}$

b) calculer $p(B)$

2\% un jeu consiste à tirer sumultannément. deux jetons du sac:

• un jeton souge apporte 2 points.
• un jeton vert n’approrte rien.
• un jeton noir fait prendre 4 points.

soit X la variable aléatoire qui prend pour valeurs le gains algébrique des points appostés par les deux jetonstirés. AELDOCUMENTS

a) justifier que les valeurs prises par X sent $-4 ;-2 ; 0 ; 2$ et 4

ماكش باش تلقى أحسن من كتب المراجعة متع WaelDocuments.com – طبعة واضحة

livraison à domicile $7 \mathrm{dt}$

WaelDocuments

\ شنوّا فيهم الكتب ؟} للكتب قبل ما تشري

*المناظرات السابقة للباكالوريا 2009-2023 (الأعوام الكل مصلّحة)

• زوروا موقعنا: www.WaelDocuments.com – أو ابعثولنا message privé ألى صفحتنا على الفايسبوك أو الانستغرام. اسم الصفحة: WaelDocuments.com – أو إتّصلوا بأرقامنا: 50404042 أو 50454040

ماكش باش تلقى أحسن من الكتب متع

• مرفوقة بالإصلاح

livraison à domicile $7 \mathrm{dt}$ للكتب قبل ما تشري

• زوروا موقعنا: www.WaelDocuments.com – أو ابعثولنا message privé على صفحتنا على الفايسبوك أو الانستغرام. اسم الصفحة: WaelDocuments.com – أو إتّصلوا بأرقامنا: 50404042 أو 50454040
  b) verrifier que $p(X=2)=\frac{2}{5}$ \%ocuemen

c) récopier et complèter le tablecu suivant:

\begin{tabular}{|c|c|c|c|c|c|}
\hline$x_{\mathbf{i}}$ & -4 & -2 & 0 & 2 & 4 \
\hline$p\left(X=x_{\mathbf{i}}\right)$ & & & & $\frac{2}{5}$ & \
\hline
\end{tabular}

d) calculer l’espérance $E(X)$ et la variance V(X).

e) ce jeu est – il favorable.

$1 \%$ a) AELDOCUMENTS

A obtenir deux jetons de méme couleur.

ou

$\operatorname{cand}(A)=\left(C_{3}^{2}+\right.$
b)

$$
p(A)=\frac{\operatorname{cand}(A)}{\operatorname{cand}(\Omega)}=\frac{\left(C_{3}^{2}+C_{2}^{2}\right)}{C_{6}^{2}}=\frac{3+1}{15}=1
$$

$B$ obtenir du moins un jeton vert»

$$
\begin{aligned}
\operatorname{cand}(B) & =\left(C_{3}^{1} \times C_{2}^{1}\right)+\left(C_{1}^{1} \times C_{2}^{1}\right)+\left(C_{2}^{2}\right) \
P(B) & =\frac{\operatorname{cand}(B)}{(B)} \
& =\frac{\left(C_{3}^{1} \times C_{2}^{1}\right)+\left(C_{1}^{1} \times C_{2}^{1}\right)+C_{2}^{2}}{C_{6}^{2}} \
& =\frac{6+2+1}{15} \
& =\frac{9}{15}
\end{aligned}
$$
$2^{\text {ème }}$ mèthode :

$$
p(B)=1-p(\bar{B})
$$

B: obtenir alcune boule verte»

$$
\operatorname{cand}(\bar{B})=\left[C_{3}^{2}+\left(C_{1}^{1} \times C_{3}^{1}\right)\right]
$$

$$
\begin{aligned}
P(B) & =1-\frac{\operatorname{card}(\bar{B})}{\operatorname{card}(\Omega)} \
& =1-\frac{\left[C_{3}^{2}+\left(C_{1}^{1} \times C_{3}^{1}\right)\right]}{C_{6}^{2}} \
& =1-\frac{6}{15} \
& =\frac{9}{15}
\end{aligned}
$$

AELDOCUMENTS
$2 \%$ d.

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد
déterminer la loi de probabilite de $X$ :

$$
\begin{aligned}
& P(\boldsymbol{X}=-4)=\frac{C_{1}^{1} \times C_{2}^{1}}{C_{6}^{2}}=\frac{1 \times 2}{15}=\frac{2}{15} \
& p(\boldsymbol{X}=-2)=\frac{C_{1}^{1} \times C_{3}^{1}}{C_{6}^{2}}=\frac{1 \times 3}{15}=\frac{3}{15} \
& p(\boldsymbol{X}=0)=\frac{C_{2}^{2}}{C_{6}^{2}}=\frac{1}{15} \
& p(\boldsymbol{X}=2)=\frac{C_{3}^{1} \times C_{2}^{1}}{C_{6}^{2}}=\frac{6}{15}=\frac{2}{5} \
& p(\boldsymbol{X}=4)=\frac{C_{3}^{2}}{C_{6}^{2}}=\frac{3}{15}
\end{aligned}
$$
c)

AELDOCUMENTS

\begin{tabular}{|c|c|c|c|c|c|}
\hline$x_{i}$ & 4 & -2 & 0 & 2 & 4 \
\hline$p\left(X_{i}=x_{i}\right)$ & $\frac{2}{15}$ & $\frac{3}{15}$ & $\frac{1}{15}$ & $\frac{6}{15}$ & $\frac{3}{15}$ \
\hline
\end{tabular}
d)

$$
\begin{aligned}
E(X)= & \left(-4 \times \frac{2}{15}\right)+\left(-2 \times \frac{3}{15}\right)+\left(0 \times \frac{1}{15}\right)+ \
& \left(2 \times \frac{6}{15}\right)+\left(4 \times \frac{3}{15}\right) \
\left.Q^{2}\right) & \frac{2}{3}
\end{aligned}
$$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

Page Fb: WaelDocuments.com

Réponse:

1) arbe ponding:

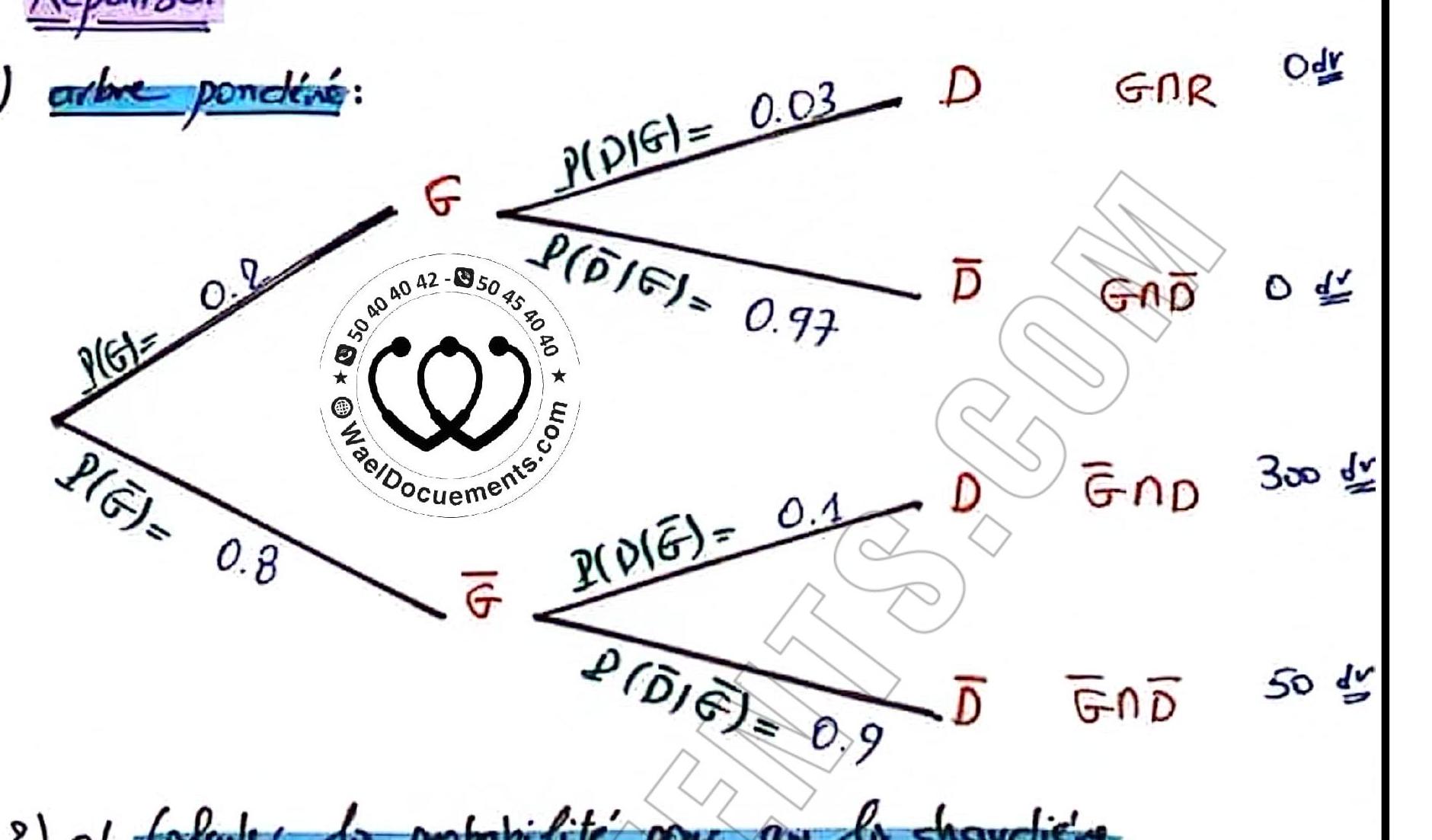
2) al $\frac{\text { Gafoutes la probabilité pour qu la chavelieine }}{G}$

$$
P(G \cap R)=P(G) \cdot P(D \mid G)=0.2 \times 0.03=0.006 \text {. }
$$
b) $\mathrm{Mq} \mathrm{P}(D)=0.086$

$$
\begin{aligned}
& P(D)=P(G) \cdot P(D \mid G)+P(\bar{G}) \cdot \mathcal{P}(D \mid \bar{G}) \
& \Rightarrow 0.2 \times 0.03+0.6 \times 0.1 \
& =0.086 \
& \text { Déduine \&( } \bar{D}) \
& 8(D)+8(\bar{D})=1
\end{aligned}
$$

$$
\begin{aligned}
& P(\bar{D})=1-P(D)=1-0.086=0.914
\end{aligned}
$$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

$$
\begin{aligned}
& P(G \mid D)=\frac{P(G \cap D)}{P(D)}=\frac{0.006}{0.086}=0.0698 \
& \quad \text { or }\left{\begin{array}{l}
P(G \cap D)=0.006 \
P(D)=0.086
\end{array} \quad(\text { question } 2 / \mathrm{a} / 6)\right.
\end{aligned}
$$

$$
\begin{aligned}
P(G \cup \bar{D}) & =P(G)+P(\bar{D})-P(G \cap \bar{D}) \
& =0.2+0.914-0.194=0.92
\end{aligned}
$$

$$
\begin{cases}P(G)=0.2 & \text { (arbore) } \ P(\bar{D})=0.914 & \text { (quertion } 2 / b) \ P(G \cap \bar{D})=P(G) & P(\bar{D} \mid G)=0.2 \times 0.97=0.194\end{cases}
$$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

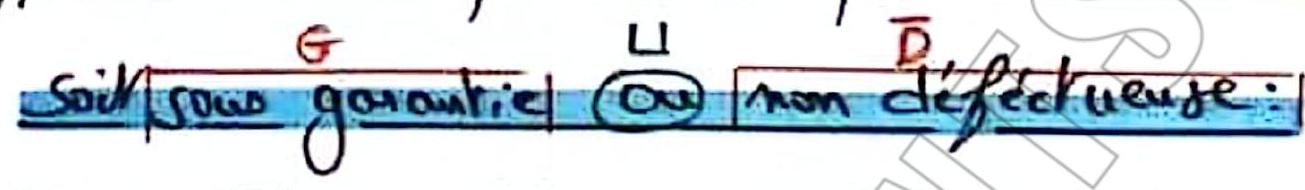
5) le conksile ou le riparation est gratuit si hor Chandière est soms garautie. ${ }^{G}$ are difout $D$

$0 \stackrel{\mathrm{dr}}{ } \sim G \cap D$ ou $G \cap \bar{D}$

il coute 50 dr si la chandiére d’eal pas sous garantie el m’estpas Défecturage.

$50 \stackrel{d V}{\sim} \sim \bar{G} \cap \bar{D}$

il coûte 300 dve si la choudiére n’at ples sous Garoutic ev difictuarge.

$300 d \sim \sim \bar{E} \cap D$

Soit $x$ le vacioble aléarioisere qui représante le coût de conriôle on reparation d’elue choudicise:
a)

$$
\begin{aligned}
& \text { a) la boi de polabilité:In } \
& X={0,50,300} \
& P(X=0)=P(G \cap D)+P(G \cap \bar{D}) \
& =0.2 \times 0.03+0.2 \times 0.97=0.2 \
& P(x=S D)=P(\bar{G} \cap \bar{D})=0.8 \times 0.9=0.72 \
& P(X=300)=P(\bar{G} \cap D)=0.8 \times 0.1=0.08 \
& E P_{i}=1 \quad \text { (Veifici) }
\end{aligned}
$$
b) Heopelcurre: le côt (moget) de conviole ou de ciponation.

$$
\begin{aligned}
E(x) & =\varepsilon x_{1} \cdot p_{i} \
& =0 \times 0.2+50 \times 0.72+300 \times 0.08 \
& =60 \quad \text { AELDOCUMENTS }
\end{aligned}
$$

a Variance: $V(n)=E n_{i} p_{i}-(E(n))^{2}$

$$
\begin{aligned}
& =0^{2} \times 0.2+50^{2} \times 0.72+300^{2} \times 0.00-(60)^{2} \
& =5400
\end{aligned}
$$

L’écond-rgpez $\sigma(x)=\sqrt{y(x)}=\sqrt{5400}=73.49$

يمكنكم شراء كتب بالوريا جميع الشعب جمبيا على صفحتناد

Raise the bar higher

$$

$$

Success

\section*{Page Fb: WaelDocuments.com}

Une entreprise vend des ordinateurs d’une certaine marque.

Le service après-vente s’aperçoit que les ordinateurs peuvent présenter deux types de défauts: l’un est lié au clavier et l’autre lié d l’affichage.

La probabilité pour qu’un ordinateur tiré au hasard de présenter un défaut de clavier est 0.1 .

En présence du défaut de clavier, la probabilité que l’ordinateur soit en panne d’affichage est 0,05 . Alors qu’en absence de défaut de clavier, la probabilité de ne pas présenter de défaut d’affichage est 0.9.

On donne les évènements suivants :

$C$ : « l’ordinateur présente un défaut de clavier»

A: « l’ordinateur présente un défaut d’affichage».

1) a- Construire un arbre pondéré décrivant cette situation.

b- Calculer $\mathrm{p}(\mathrm{A})$

2) Cette entreprise a fait une offre de vente gornme suit :

• 400 DT pour un ordinateur quilimésenteles dey défauts.
• 500 DT pour un ordinateur quiprésentesellement le défaut $A$.
• 600 DT pour un ordinateur qui présente seulement le đéfaut $C$.

• 700 DT pour un ordinateun quine presente oucup défgirt

Soit $X$ la variable aléátoje qư indique le montant payé par un client qui achète un ordinateur avec cette offres

a-Montrer que $p(x=700)=0,81$

b-Déterminer loloide probabilité de $x$.

$c$-Calculer l’espérance mathématique de $x$

3)Un groupe de 10 clients vont d̀ ce magasin. On note par $y$ la variable aléatoire donnant le nombre des clients qui achète un ordinateur avec 7000T.

a- Donner la loi de probabilité de $y$.

b- Déterminer la probabilité pour que deux clients achètent un ordinateur avec 7000 T.

c- Déterminer la probabilité pour qưau moins un client achète un ordinateur avec 7000 T.

d- Déterminer la probabilité pour qu’au plus un client achète un ordinateur avec 7000 T.

e- Calculer l’espérance $E(Y)$ et la variance $V(Y)$.

\section*{AELDOCUMENTS}

.com

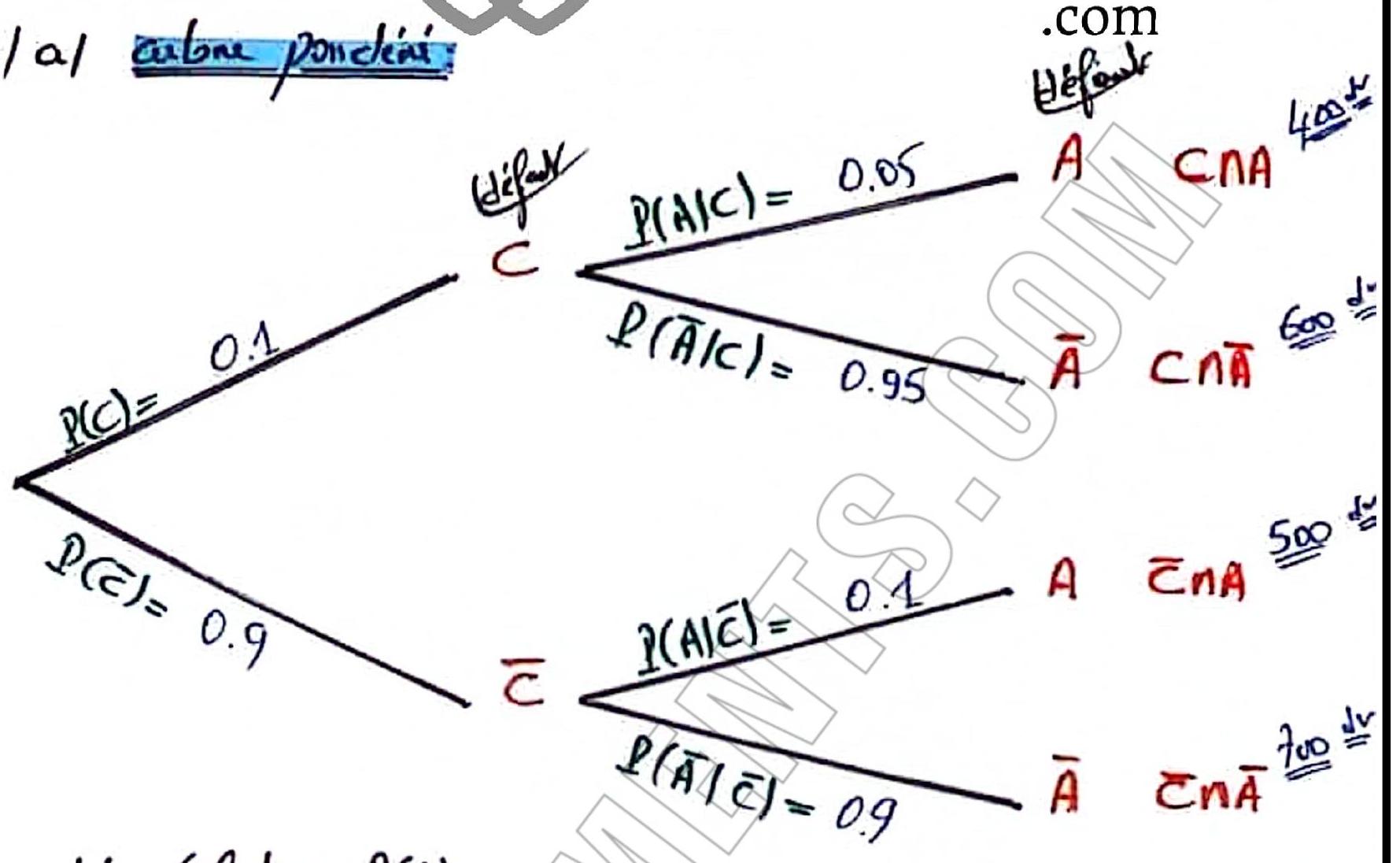
b) Golartes $P(A)$

$$
\begin{aligned}
P(A) & =P(C) \cdot 8(A \mid C)+P(\bar{C}) \cdot P(A \mid \bar{C}) \
& =0.1 \times 0.05+0.9 \times 0.1 \
& =0.095
\end{aligned}
$$

2/0 $400 \frac{\text { Nv }}{\text { pour un od gui puisante leo } 2 \text { diffar }}$

L. ordinatou qui pisante le $\frac{\text { difour Cl eV le difarta }}{C n A}$

(eV) presante pas le difor $C$. $\overline{-}$

$\bar{c} \cap A$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

• 600 dr poin un odinotan qui pésante senlineur le diffarc. Lo adinatanir qui prisant the difatr $C$ c|

(eV) paéjante pas le difout ${ }^{\bar{A}} \bar{A}$ !

$\rightarrow$ ardinatem quar ies piessante pas le difinatr $C$

(eV) me puisante pas le difark A.

$x$ lo racizble aliokive qui widique le montant payé par unclient qui adrite un adinaraun.

$$
\text { al } \begin{aligned}
P(x=700)=P(x=700)=0.81 \
\begin{aligned}
P(\bar{C} \cap \bar{A})) & =P(\bar{C} \mid \cdot P(\bar{A} \mid c) \
& =0.9 \times 0.9=0.81 .
\end{aligned}
\end{aligned}
$$

b) doi de probabilité:

AELDOCUMENTS

$$
x={400,500,600,700}
$$

$$
{ }_{0} P(x=4(0)=P(C \cap A)=P(C) . P(A \mid C)=0.1 \times 0.05=0.005 .
$$

(1) $P(x=500)=P(\bar{C} \cap A)=P(\bar{C}) \cdot R(A \mid \bar{C})=0.9 \times 0.1=0.09$

0 $R(X=600)=R(C \cap \bar{A})=R(C) \cdot R(\bar{A} \mid C)=0.1 \times 0.9 .5=0.095$.

(1) $P\left(X=7_{00}\right)=R(\bar{C} \cap \bar{A})=R(\bar{C}) .8(\bar{A} / \bar{C})=0.9 \times 0.9=0.81$

$$
\sum p_{i}=1 \quad \frac{\text { (Virificel }}{2}
$$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد
c) Cetermina l’espéfances te monvant mogen payé pas im client qui achite un adiacham.

$$
\begin{aligned}
E(x) & =\varepsilon \eta_{i} \cdot p_{i} \
& =400 \times 0.001+500 \times 0.09+600 \times 0.095+700 \times 0.81 \
& =671 .
\end{aligned}
$$

parmétue $n=10$

3) Un group de 10 clienty vont a’ ce magasin y la Variable aliatoive clonnaut la mbue che clienV qui accile on adinaliou arre 700 \&y -Succés $p=p(x=700$, a) toi de probabilite: $=0.81$

y sair une loi binomiale AELDOCUMENTS de probabilité noté $p=p(x=700)=0.81$ .com

$$
\bar{p}=1-p=1-0.81=0.19
$$

de paramérie $n=10$

$$
p(y=k)=C_{n}^{k}-p^{k} \cdot \bar{p}^{n-k}
$$

$$
p(y=k)=C_{10}^{k} \cdot(0.81)^{k} \cdot(0.19)^{10-k}
$$

avec $k \in{0,1,2, \ldots 10}$
b) Détermines la probabilit’ pon que cleux clicuts adiete cm ordinatoun avec $7_{00} \mathrm{Jv}$.

$$
\begin{aligned}
& p(y=2)=C_{10}^{2} . \
& (0.81)^{2} . \
& (0.19)^{10-2} 0^{1000^{1002-090}} \
& =C_{\infty}^{2} \cdot(0.81)^{2} \cdot(0.19)^{8} \
& =0.00005
\end{aligned}
$$

c) Déterminer la probabilite’ qu » ou moins im client adúte un adinatem avec 700 $10 y=1$ ou $y=2$…. on $y=10$

$$
\begin{aligned}
& y=1 \text { on } y=2 \text { on } y=3 \quad \cdots \text { on } y=10 \
& \oplus \
& \text { AELDOCUMENTS } \
& p(y \geqslant 1)=1-p(y=0) \
& { }^{000042-0^{50_{85}} B_{0}}=1\left(C_{10}^{0} \cdot(0.81)^{0} \cdot(0.19)^{10-0}\right) \
& =1-\left(C_{12}^{0} \cdot(0.81)^{0} \cdot(0.19)^{10}\right) \
& =0.99
\end{aligned}
$$

$$
\begin{aligned}
& =1 \
& \text { NTS } \
& \text {.com }
\end{aligned}
$$

d/ Détermines la paobabilik’ poun gaximum 1 y av plus con clienV a chite un adinathan avec 700 dr. $y=0$ ou $y=1$

$$
\begin{aligned}
p(y \leqslant 1) & =p(y=0)+p(y=1) \
& =C_{10}^{0} \cdot(0.81)^{0} \cdot(0.19)^{10-0}+C_{10}^{1} \cdot(0.81)^{1} \cdot(0.19)^{10-1} \
& =C_{10}^{0} \cdot(0.81)^{0} \cdot(0.19)^{10}+C_{10}^{1} \cdot(0.81)^{1} \cdot(0.19)^{9} \
& =0.000002
\end{aligned}
$$

تابعونا على صفحتنا في الفايسبوك باش تلقى تلاخيص في جميع المواد

$$
E(x)=n \cdot \mathbb{P}=10 \times 0.81=8.1
$$

taUariance:

$$
V(x)=n \cdot p \cdot \bar{p}=10 \times 0.81 \times 0.19=1.539
$$

oubien

$$
V(x)=E(x) \cdot \Phi=8.1 \times 0.19=1.539
$$

teecand =ryper

$$
\sigma(x)=\sqrt{V(x)}=\sqrt{1.539}=1.24
$$

يمكنكم شراء كتب مراجعة باكالوريا جميع الشعب جميع المواد حصريّيا على صفحتنا

ماكش باش تلقى أحسن من كتب المراجعة متع WaelDocuments.com – طبعة واضحة

livraison à domicile $7 \mathrm{dt}$

WaelDocuments

\ شنوّا فيهم الكتب ؟} للكتب قبل ما تشري

*المناظرات السابقة للباكالوريا 2009-2023 (الأعوام الكل مصلّحة)

• زوروا موقعنا: www.WaelDocuments.com – أو ابعثولنا message privé ألى صفحتنا على الفايسبوك أو الانستغرام. اسم الصفحة: WaelDocuments.com – أو إتّصلوا بأرقامنا: 50404042 أو 50454040

ماكش باش تلقى أحسن من الكتب متع

• مرفوقة بالإصلاح

livraison à domicile $7 \mathrm{dt}$ للكتب قبل ما تشري

• زوروا موقعنا: www.WaelDocuments.com – أو ابعثولنا message privé على صفحتنا على الفايسبوك أو الانستغرام. اسم الصفحة: WaelDocuments.com – أو إ